Understanding Right Angle Trigonometry
For a woodworker, being able to "solve" right triangles is an extremely important skill. Compound miters, multi-sided structures and a variety of other complex building projects can all be understood and calculated using right triangle trigonometry. If the term trigonometry causes you to suffer a sudden onset of "math anxiety," you'll be happy to know that the trigonometry you need for woodworking isn't all that complicated.
A basic knowledge is all that's necessary to solve just about any angle problem that will ever come up in woodworking. Below, we'll reproduce a few well-know formulas and relationships from right triangle trigonometry that can really come in handy in woodworking.
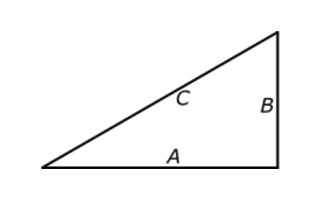
The Pythagorean Theorem
The Pythagorean Theorem is a relation in geometry between the length of the three sides of a right triangle:
For any right triangle ABC, A² + B² = C² where A and B are the sides of the triangle that meet at a 90 degree angle. Example: Using the Pythagorean Theorem to determine the length (L) of a diagonal brace that projects 8'' out from the surface of a wall to support a shelf, and 12'' down from the bottom surface of the shelf :
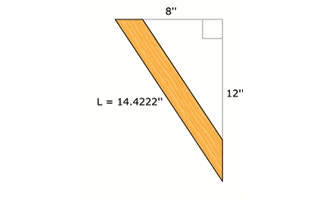
L² = 8² + 12²
=> L² = 208
=> sqrt(L²) = sqrt(208)
=> L = 14.4222''
=> L = (approx.) 14-27/64''
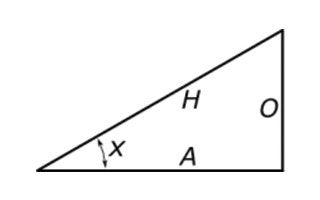
The Trigonometric Functions Sine, Cosine and Tangent
The Pythagorean Theorem comes in handy for calculating the dimensions of the sides of right triangles, but for angle calculations, it's necessary to use trigonometric functions. Trigonometric functions describe the relationships between the sides of a right triangle: Sin(x) = O/H Cos(x) = A/H Tan(x) = O/A
The relationships apply to all right triangles regardless of the size or proportions of the triangle.
Example: Above, we used the Pythagorean Theorem to calculate the length of a diagonal shelf brace based on the 8'' projection of the brace out from the wall, and the 12'' projection of the brace down from the bottom of the shelf. This time, instead of having the brace meet the wall at exactly 12'' below the shelf, we want the diagonal brace to tilt out from the wall at a 30 degree angle, but still project exactly 8'' out from the wall.
Because we know angle w (where the wall and the brace meet) and the length of the side of the right triangle formed by the brace, the wall and the bottom of the shelf opposite angle w, we can use the sine function to determine the length (L) of the brace:
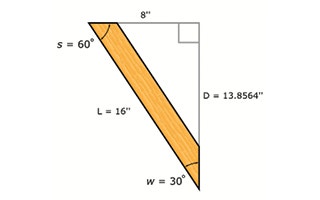
sin(w) = 8/L
=> sin(30) * L = 8
=> .5 * L = 8
=> L = 8/.5
=> L = 16''
If we also needed to know the distance (D) down from the bottom surface of the shelf to the bottom tip of the brace, we could use either the Pythagorean Theorem: D = sqrt(16² - 8²) = 13.8564 or the tangent function: D = 8/tan(30) = 13.8564 = approximately 13-57/64''
The angle s of the cut where the brace meets the shelf is simply the complement of angle w: 90 - 30 = 60 degrees
Calculating Trigonometric Function Values
To calculate the value of trigonometric function, you either need to use a scientific calculator or a table of trigonometric function values. A scientific calculator is a handy tool to have around the shop and worth the expense of adding to your tool collection. You'll also find tables of trigonometric values on the Rockler website at Woodworking Math Formulas, Tables and Calculators.
Inverse Trigonometric Functions
In some cases, the value of the trigonometric function is the known quantity, and the angle that it corresponds to needs to be determined. In those cases it is necessary to use the inverse trigonometric functions. Inverse trigonometric functions are, simply stated, trigonometric functions in reverse. In other words, they calculate the angle that corresponds to a given trigonometric function value.
The inverse of the trigonometric functions are often referred to as the "arc" function - "arc sine," for example, refers to the inverse function of the sine function. For the purposes of making woodworking, the inverse trigonometric functions can be defined: x = arcsin[sin(x)] x = arccos[cos(x)] x = arctan[tan(x)] For example, sin(30) = .5 arcsin(.5) = 30 arcsin[sin(30)] = 30 sin[arcsin(.5)] = .5
Example: Above, we used the Pythagorean Theorem to calculate the length of a diagonal brace that projects 8'' out from a wall to support a shelf, and 12'' down from the bottom of the shelf. The Pythagorean Theorem told us the length of the brace (14.4222'') but nothing about the angles we need to cut to fit the brace against the bottom of the shelf, and up against the wall. We'll use the inverse trigonometric functions to find the angle s of the cut at the shelf end of the brace and the angle w of the cut where the brace will meet the wall.
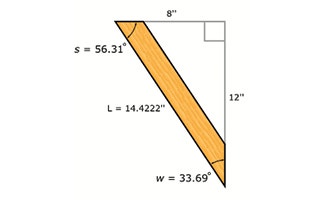
tan(w) = 8/12
=> arctan[tan(w)] = arctan(8/12)
=> w = arctan(.0667)
=> w = 33.69 degrees
Alternatively, we could have used either the arc sine and sine functions with the 14.4222'' length of the diagonal brace and the 8'' projection of the brace out from the wall, or similarly, the arc cosine and cosine function with the length of the brace and the 12'' projection of the brace down from the shelf.
The angle s for the cut at the end of the brace that meets the shelf could also be calculated using inverse trigonometric functions, but it's easier to simply subtract angle w from 90 degrees to find its complement: 90 degrees - 33.69 degrees = 56.31 degrees = angle s
Keep the inspiration coming!
Subscribe to our newsletter for more woodworking tips and tricks